Please keep in mind that this is all theoretical. It does not account for line weakening due to knots, damage, or the walker bouncing on the line. It also ignores the presence of slings. Generally, you can assume that "Length" refers to the span of webbing between the two end slings. This is the ideal case where the line is perfectly attached to the end points, and the walker is standing stationary in the middle. Take the calculated tension as a general guideline rather than absolute fact for the sake of safety.

The solutions below are independent of the webbing's material properties. This is very handy, because those properties change a little with each manufacturing run, long-term use, exposure ot the elements, and the type of webbing. However, given that webbing can only carry tension and nothing else, we can determine the line's tension with only a couple pieces of information; pieces of information that are easily measured by anyone!

To begin, we have a diagram of the "ideal" slackline. Its horizontal span is length L, the diagonal line length is D, the center-dip is of height H, and the slacker's presence poses force W on the middle of the line. In English units, one's weight is this force. If you use the Metric/SI system, your mass in kilograms must be multiplied by the acceleration we experience due to gravity. So, to get your mass in kilograms converted to kiloNewtons on the surface of our fair Earth, take your mass in kilograms, multiply by 9.81, and then divide by 1000. This will get you your weight in kiloNewtons! Of course, if you live on the moon, or perhaps the top of Mt. Everest, this would require a different value than 9.81!

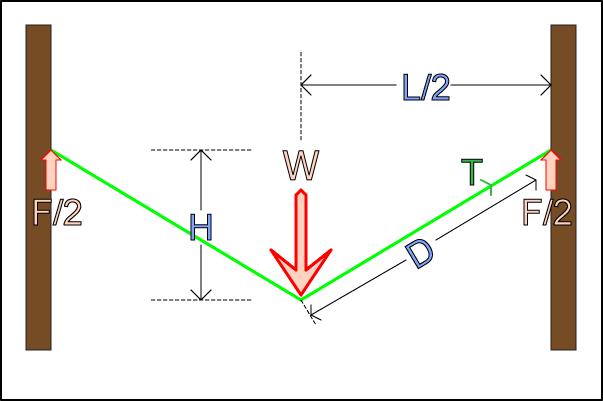
The vertical reaction forces at the ends are each equal to half of the vertical force imposed by the slacker. We will not be concerned with the horizontal component of the reaction at the ends in the calculation of the line tension (which consequently, is made of the vertical and horizontal components). The next image shows a triangle, composed of the various forces and distances we will be concerned with. I will solve for the tension with two different methods. The first one is the simplest (and is also the formula used in the calculator above), and the second is almost as much so.

In the diagram above, forces are green and lengths are in blue. While they may not have the same units, they are all proportional to each other with respect to the triangle they form.

Solving - First Method (Pythagorean Theorem)

So, solving with the first method, we develop a simple relation. The ratio of the total stretched line length to the vertical displacement in the middle of the line is equal to the ratio of the tension in the line to the vertical component of that tension (the vertical reaction force at one end). This relation is shown in Equation 1.

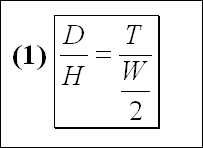
Next, we use the ever-handy Pythagorean Theorem to determine the distance D. We already know H and L/2 (L/2 being half of the total horizontal length L). According to the theorem, the longest side of a right triangle (the hypotenuse) is equal to the root of the sum of the squares of the other two, shorter sides. Words are nice, but pictures and simple equations are nicer. See Equation 2 for a clearer idea.

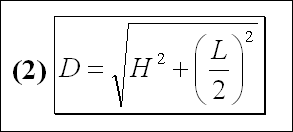
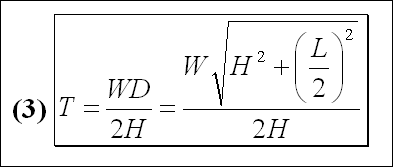
In the words of one of my college engineering professors that I will never forget, "two equations, two unknowns, DONE!" Right now, we have all we need to solve this out for the tension, T. Continuing, we take Equation 1 and re-arrange the ratios so that we have a relation for the tension, T. Then, we substitute Equation 2 into it for the third part (take D in the middle part and replace it with Equation 2). DONE!

Solving - Second Method (Trigonometric Identities)

Again, we return to the triangle diagram with forces and lengths. This time, we will employ different a different method to determine the line's tension. We begin by solving for the angle denoted in the second diagram, Theta (the "O" with the line in the middle...the symbol won't display properly in most browsers). The tangent of an angle is equal to the ratio of the side opposite the angle to the side adjacent to the angle (not the hypotenuse). Remember, the trigonometric identities (sine, cosine, tangent, etc.) only work on right triangles, a.k.a. triangles with a 90 degree, or "square" corner. Anyway, the inverse, or arctangent, of this ratio of sides equals the angle between them. Some calculators want angles in degrees, other in radians. Make sure to check before doing things yourself! One degree = pi/180 radians. Without further ado, Equation 4 shows the calculation for the angle Theta.

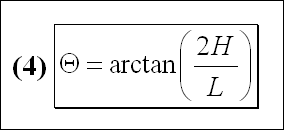
The sine of an angle is equal to the ratio of the opposite side of the triangle to the hypotenuse of the triangle. This means that sine(Theta) = (W/2)/T. Rearranging this, we get the beginning of Equation 5. We then substitute Equation 4 for the value of Theta in Equation 5, and there we have our answer. The angle will be the same for the triangle formed by the lengths and that formed by the vectors of the forces involved. This method will yield exactly the same answers as the first method.